Torque
Обзор
Source: Nicholas Timmons, Asantha Cooray, PhD, Department of Physics & Astronomy, School of Physical Sciences, University of California, Irvine, CA
The goal of this experiment is to understand the components of torque and to balance multiple torques in a system to achieve equilibrium. Much like how a force causes linear acceleration, torque is a force that causes a rotational acceleration. It is defined as the product of a force and the distance of the force from the axis of rotation. If the sum of the torques on a system is equal to zero, the system will not have any angular acceleration.
Принципы
Torque is defined as the cross product of the distance, r, from the axis of rotation at which a force is applied, and the force, F:
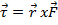 , (Equation 1)
, (Equation 1)
where 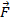 is the applied force and
is the applied force and 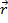 is the distance to the axis of rotation. Torque has units of force multiplied by distance and so is measured in Newton meters. Because torque is a vector, it has both magnitude and direction. The direction of the torque is perpendicular to the plane made by the force and distance components. The direction can be determined using one's right hand. Extend the pointer finger in the direction of the first component. Extend the middle finger in the direction of the second component. Once this is done, the direction of the extended thumb is the direction of the torque. An example is a wrench tightening a bolt. A force is applied at the end of the wrench, some distance from the bolt, which provides a torque to rotate the bolt into place. The longer the distance
is the distance to the axis of rotation. Torque has units of force multiplied by distance and so is measured in Newton meters. Because torque is a vector, it has both magnitude and direction. The direction of the torque is perpendicular to the plane made by the force and distance components. The direction can be determined using one's right hand. Extend the pointer finger in the direction of the first component. Extend the middle finger in the direction of the second component. Once this is done, the direction of the extended thumb is the direction of the torque. An example is a wrench tightening a bolt. A force is applied at the end of the wrench, some distance from the bolt, which provides a torque to rotate the bolt into place. The longer the distance 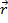 , the larger the torque, as can be seen from Equation 1. The force needed to rotate an object can be reduced significantly by simply increasing the length of the force to the axis of rotation.
, the larger the torque, as can be seen from Equation 1. The force needed to rotate an object can be reduced significantly by simply increasing the length of the force to the axis of rotation.
A torque on a system will cause an angular acceleration on that system:
 . (Equation 2)
. (Equation 2)
Here,  is angular acceleration and
is angular acceleration and  is the moment of inertia for that system. This is the rotational equivalent of Newton's second law,
is the moment of inertia for that system. This is the rotational equivalent of Newton's second law, 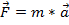 , with mass replaced with the moment of inertia and acceleration replaced with angular acceleration.
, with mass replaced with the moment of inertia and acceleration replaced with angular acceleration.
This experiment will include a meter stick that is able to rotate freely about its axis, as shown in Figure 1.

Figure 1: Experimental setup.
Weights are attached at various distances from the axis of rotation, which will cause a torque on the system. If the torques on both sides are balanced, the meter stick should not rotate from rest. To examine the torque from a weight or combination of weights, a force scale can be attached to the other side. The force the scale reads multiplied by the distance from the scale to the axis of rotation will be equal to the torque from the weights.
Процедура
1. Using two weights to balance the beam.
- Start by connecting one 200-g weight to the first hook on the right. Then, connect a 200-g weight to the first hole on the left. If released from rest, the beam should not rotate.
- Remove the 200-g weight from the left side. Determine using Equation 1 where a 100-g weight would need to be placed to balance the torque from the right side. Place the weight and confirm the prediction.
2. Using three weights to balance the beam.
- Connect a 100-g weight to the first hook on the right. Place a 100-g weight on the third hook to the right.
- Determine where to place a 200-g weight on the left side to balance the torques.
- Determine where to place a 100-g weight on the left side to balance the torques.
3. Using multiple weights to balance the beam.
- Connect a 200-g weight to the fourth hook on the right side.
- Using any combination of 100-g and 200-g weights find three ways in which the torque from the right side can be balanced on the left side.
- With the 200-g weight still connected to the fourth hook on the right side, connect a force scale to the first hook on the left side and pull until it is in equilibrium. Make sure to keep the force scale perpendicular to the beam. Record the force. Do this for each hook on the left side and record the values.
- With the 200-g weight still connected to the fourth hook on the right side, use a protractor to rotate the beam 30°. Attach the force scale to the third hook on the left and record the force. Repeat for 60°.
Результаты
Step 1.2: Connect a 100-g weight to the second hole on the left.
Step 2.2: Connect the 200-g weight to the second hole on the left.
Step 2.3: Connect the 100-g weight to the fourth hole on the left.
Step 3.2: There are six different ways:
1) 200 g - 4th hole
2) 200 g - 1st hole, 200 g - 3rd hole
3) 100 g - 2nd hole, 200 g - 3rd hole
4) 100 g - 1st hole, 200 g - 2nd hole, 100 g - 3rd hole
5) 200 g - 2nd hole, 100 g - 4th hole
6) 100 g - 1st hole, 100 g - 3rd hole, 100 g - 4th hole
Table 1. Results for steps 3.3 and 3.4.
| Hook # | Force
(N) |
Force at 30°
(N) |
Force at 60°
(N) |
| 1 | 8 | - | - |
| 2 | 4 | - | - |
| 3 | 2.7 | 2.3 | 1.3 |
| 4 | 2 | - | - |
These results confirm the predictions made by Equation 1. Each weight connected to the beam provides a torque on the system. While weights on one side cause a torque in one direction, weights on the other side cause a torque in the opposite direction. According to Equation 2, when the sum of the torques on the beam is equal to zero, the beam will not rotate when released from rest. In every part of the experiment, when the beam is in equilibrium, the torques must add up to zero.
Заявка и Краткое содержание
As mentioned earlier, a simple application of torque is using a wrench to tighten a bolt. The important thing to remember is that torque has two components. If it is difficult to tighten a bolt with the wrench in hand, a worker has two options. He can either apply more force or just get a longer wrench. Usually, the latter is the easier choice.
When a car commercial quotes some value of torque, it is a good idea to pay attention. As can be seen by the equation  , torque is what makes the wheels on a car accelerate. The more torque, the more acceleration.
, torque is what makes the wheels on a car accelerate. The more torque, the more acceleration.
A seesaw on the playground is a perfect application of torque. The beam rotates about the fulcrum, and the torque is provided by the people sitting on either end. If one person has more mass, then the torque on that side will be larger and the person on the other side will be lifted up. To get that person down, the person on the ground provides a torque by pushing up with his legs to counter the force of his, weight and he is in turn lifted up.
In this experiment, the two main components of torque were examined. Torque is the product of a force and the distance between the force and an axis of rotation. By placing different weights at different positions on a rotating beam, varying quantities of torque were created. The heavier weight corresponded to a larger force and therefore a larger torque. Placing weights further from the axis of rotation created a larger lever arm, which resulted in a larger torque than if the same weight had been placed closer to the axis of rotation. When the total torque on the beam was equal to zero, the system was in equilibrium.
Перейти к...
Видео из этой коллекции:

Now Playing
Torque
Physics I
26.2K Просмотры

Newton's Laws of Motion
Physics I
76.9K Просмотры

Force and Acceleration
Physics I
79.6K Просмотры

Vectors in Multiple Directions
Physics I
182.7K Просмотры

Kinematics and Projectile Motion
Physics I
73.1K Просмотры

Newton's Law of Universal Gravitation
Physics I
192.7K Просмотры

Conservation of Momentum
Physics I
43.6K Просмотры

Friction
Physics I
53.1K Просмотры

Hooke's Law and Simple Harmonic Motion
Physics I
61.6K Просмотры

Equilibrium and Free-body Diagrams
Physics I
37.6K Просмотры

Rotational Inertia
Physics I
43.8K Просмотры

Angular Momentum
Physics I
36.6K Просмотры

Energy and Work
Physics I
50.4K Просмотры

Enthalpy
Physics I
60.6K Просмотры

Entropy
Physics I
17.8K Просмотры
Авторские права © 2025 MyJoVE Corporation. Все права защищены