Testing the Heat Transfer Efficiency of a Finned-tube Heat Exchanger

Heat exchangers transfer heat between two or more fluids. The exchangers use fluid species which flow in a separate space from an opposing stream that is providing heat. Fins can be added to the flow area to facilitate more heat transfer, as they increase the surface area available for transference. The added fins decrease the area through which the species flows and provide more surfaces on which boundary layers can form, resulting in flow that is less turbulent. The less turbulent a flow, the larger boundary layer it will have. A boundary layer inhibits heat transfer, so with less turbulent flow less heat is transferred. When the boundary layer is laminar, there is very little mixing.

The relationship between the area through which heat can flow and the heat transfer coefficient is used in calculating the total heat transferred. This relationship is calculated through Equation 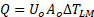1:

                   (1)

where Q is heat transferred (Btu/hr), U is overall heat transfer coefficient, A is area through which heat is transferred (ft²), ΔT_LM is the logarithmic mean temperature difference.

The overall heat transfer coefficient equation is:

                               (2)

where A_b is the surface area of bare inner pipe, A_f is the surface area of the fins, A_LM is the logarithmic mean area difference, A is the surface area of the pipe (o = outside, i = inside), Δx thickness of the pipe, k is thermal conductivity of the pipe, h = Individual heat transfer coefficient. (o=outside, i=inside)

The Wilson plot method uses experimental data to find U_oA_o from typical energy balance on the MEG flow and plot its reciprocal to 1/Re^0.8 of the inside pipe. By fitting a straight line and finding the y-intercept, which is related to the heat transfer coefficient and is described in the first two terms on the right of the equation above. A typical longitudinal rectangular profile fin efficiency equation is used as the second equation to solve for the heat transfer coefficient and fin efficiency by minimizing the sum of squares of an objective function. This method is applied to MEG flow conditions with varying water flow rates.

To calculate the heat transfer coefficient, the Reynolds Number is used, which is given by the following equation:

                                  (3)

where G is the mass velocity of fluid flow, D is the diameter of pipe where the fluid flows (D_eq, the equivalent diameter will replace D for calculations with fins), and µ is the fluid's viscosity. Fin efficiency equation for a longitudinal rectangular profile fin is:

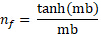                            (4)

where m is √(2h/kt), h is the heat transfer coefficient, k is thermal conductivity of the pipe, t is thickness of fin, and b is the height of fin.

The finned tube heat exchanger did not reach turbulent flow (Figure 2). The fins provide additional surfaces on which boundary layers form, as known through laminar and turbulent flow theory. If the fluid is not at a sufficient velocity, the fluid will not reach turbulence. The boundary layers between the fins overlap in the laminar region, so the fluid will remain laminar.

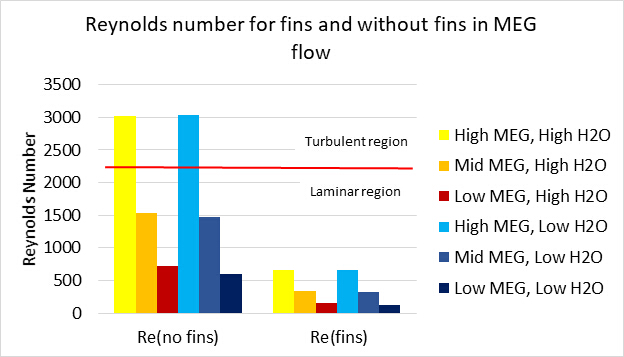
Figure 2: Reynolds numbers for each setting.

The amount of heat transferred, Q, in the tubes with and without fins at different flow rates of MEG was compared (Figure 3). The results show that a finned-tube transfers more heat than a tube without fins at the same operating conditions. In this experiment, the fins clearly improved heat transfer. This is because heat transfer is more effective when there is a greater surface area available. The finned-tube heat exchanger transferred more heat (Figure 3), despite the lower Reynolds number (Figure 2).

Figure 3: Heat transferred between exchangers with and without fins at each flow rate.

Heat exchangers are used in a variety of industries, including agriculture, chemical production, and HVAC. The goal for this experiment was to test the heat transfer efficiency of a finned-tube heat exchanger and compare it to the theoretical efficiency of a heat exchanger without fins. Experimental data was measured for three different flow rates of monoethylene glycol (MEG) and two unique water flow rates for each MEG flow rate used. The Reynold's number was determined for flow with and without the fins and was used to calculate the heat transfer coefficient, surface area, and fin efficiency for each unique trial run. This data was used to evaluate if turbulent flow is possible without the fins and under which set of trial conditions the most heat transfer occurs. The finned tubes did not reach turbulent flow. The results showed that a fin tube will transfer more heat than a tube without fins at the same operating conditions because the flow of MEG through the heat exchanger will not reach turbulence.

In the agriculture industry, heat exchangers are used in the processing of sugar and ethanol². Both of these products are processed into a juice, which must be heated to be further processed². Heat exchangers are used in heating the juices for clarification². Once the juices have been processed into even syrups, further heating with exchangers is necessary to continue processing and form molasses². Molasses is cooled using heat exchangers, after which it can be stored for later processing².

Heating, ventilation, and air conditioning systems, together known as HVAC, all make use of heat exchangers³. Household air conditioning and heating units make use of heat exchangers³. In larger settings, chemical plants, hospitals, and transportation centers all make use of similar heat exchanger HVAC, on a much larger scale³. In the chemical industry, heat exchangers are used for heating and cooling a large variety of processes⁴. Fermentation, distillation, and fragmentation all make use of heat exchangers⁴. Even more processes like rectification and purification require heat exchangers⁴.