Determining the Empirical Formula
Overview
Source: Laboratory of Dr. Neal Abrams - SUNY College of Environmental Science and Forestry
Determining the chemical formula of a compound is at the heart of what chemists do in the laboratory every day. Many tools are available to aid in this determination, but one of the simplest (and most accurate) is the determination of the empirical formula. Why is this useful? Because of the law of conservation of mass, any reaction can be followed gravimetrically, or by change in mass. The empirical formula provides the smallest whole-number ratio among elements (or compounds) within a molecular compound. In this experiment, gravimetric analysis will be used to determine the empirical formula of copper chloride hydrate, CuxCly·nH2O.
Principles
Hydrates are chemical compounds that have water molecules attached (but not covalently bonded) to the compound. Formulas that are hydrated are symbolized by a dot ("·") between the compound and the water molecule. Hydrates easily lose water molecules upon heating, leaving behind the anhydrous (without water) compound. In this case, it would be copper chloride, CuxCly. The difference in mass between the anhydrous and hydrated forms of the salt corresponds to the mass (and moles) of water in the chemical compound CuxCly·nH2O. The anhydrous copper chloride is then dissolved in water, and copper is removed through a redox reaction with aluminum to form solid copper. The difference in mass between the total copper chloride hydrate and the sum of the reduced copper metal and water molecules corresponds to the mass of chloride in the sample. The mass of each component (Cu, Cl, H2O) is converted to moles, and the mole ratio is used to determine the empirical formula of the compound. The true chemical formula of the compound cannot be determined without knowing its molecular mass, but the ratio will always remain the same.
Procedure
1. Dehydrating the Hydrate
- Accurately weigh a sample of copper chloride hydrate and place it into a pre-dried and tared crucible. It is important that the crucible is dried above 120 °C to drive off any adsorbed moisture. Typically, 1–2 g of compound will suffice.
- Heat the sample using a Bunsen burner or other flame source until it changes color from greenish-blue to a reddish-brown (Figure 1). This color change is indicative of the anhydrous form of copper chloride. The cover can stay on the crucible to prevent splattering, but should be opened slightly to allow water vapor to escape.
- Stir the sample to be sure the water is driven off the entire sample and the color is constant throughout.
- As an alternative, the sample can be placed into a drying oven above 110 °C.
- Cool the sample in a desiccator. This prevents water from rehydrating the sample.
- Measure the mass of the anhydrous sample. The difference corresponds to the water from the hydrate that was lost upon heating.

Figure 1. Bunsen burner with ceramic crucible.
2. Isolating Copper
- Transfer the sample to a 100 mL beaker and dissolve the sample in 50 mL of deionized water. The solution should turn blue once again, typically more blue than the hydrated solid.
- Add a small quantity (~0.20 g) of aluminum metal to the beaker. This will cause the copper to reduce to a reddish metal, and the aluminum will oxidize to colorless Al3+. The blue color of the solution should disappear as the Cu2+ ions form Cu0. After 30 min, add additional small pieces of aluminum to ensure all of the copper is reduced to solid copper.
- The solution now contains Al3+ ions, solid copper, and a small amount of solid aluminum.
- Dissolve any excess aluminum by adding ~5 mL of 6 M HCl. Aluminum is amphoteric, meaning it can react and dissolve in the presence of an acid or a base.
- Vacuum filter the colorless solution in a Büchner funnel containing a pre-weighed piece of filter paper. Rinse with absolute ethanol. Air dry (not oven dry) the sample to prevent the formation of copper (II) oxide.
- Measure the mass of the copper solid to determine the mass of the chloride ion by difference.
3. Calculations
- Determine the mass of the chloride ion by difference:
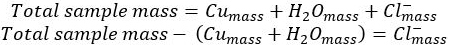
- Use the molar mass of each component of the compound to determine the moles of each component.
- Divide the moles of each component by the moles of the smallest component to give the smallest whole-number ratio of components, also known as the empirical formula of the compound.
Results
- Experiment
- Heat 1.25 g of copper chloride hydrate in a crucible. After heating and then cooling, the final mass is 0.986 g of copper chloride, CuxCly.
- Dissolve the CuxCly sample in 50 mL of deionized water and add 0.2 g of fine aluminum mesh to the beaker.
- After reacting and dissolving the excess aluminum, 0.198 g of dried copper metal is recovered.
- Subtract the mass of both copper and water from the initial copper chloride hydrate to yield the mass of chloride ion in the sample:
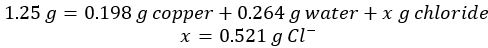
- Data
- To determine the smallest whole-number ratio of components in the compound, convert the mass of each component to moles and then divide each by the smallest number of moles in the sample (copper in this case):
Component Mass (g) Molar mass (g/mol) Moles Ratio Calculated whole-number ratio Copper 0.479 63.55 7.53 x 10-3 
1 Chloride 0.533 35.45 1.50 x 10-2 
1.99 ≈ 2 Water 0.273 18.01 1.51 x 10-2 
2.01 ≈ 2 Table 1. Experimental results.
- The resulting smallest whole-number ratio yields a formula of CuCl2·2H2O.
- In the event the final ratio yields decimal values, the whole formula would be multiplied by a constant to give whole-number values. Common fractional values are 0.25, 0.333, 0.50, 0.667, and 0.75. For example, if a smallest whole-number ratio yielded the formula yielded C7H9NO2.5, the entire formula would be multiplied by 2 to give the empirical formula C14H18N2O5.
- A molecular formula cannot be determined from the empirical formula without knowing the molecular mass of the compound. The reason for this is demonstrated in the example below:
Name Molecular Formula Empirical Formula Acetic acid CH3COOH CH2O Formaldehyde CH2O CH2O Glucose C6H12O6 CH2O Table 2. Example of a common empirical formula.
All three compounds have the same empirical formula, but very different molecular formulas.
Application and Summary
In one example, suppose an unknown biomolecule containing only C, H, and O is found to act well as a new fuel. One way to determine the formula of the fuel would be to combust it in air and analyze the products:
CxHyOz + O2 → mCO2 + nH2O
While O2 is in excess, we would know all the carbon in CO2 originated from the biomolecule and all the hydrogen would be present in H2O. The difference between that total mass and the mass of initial sample would be the mass of oxygen in the molecule. We could then convert to moles and determine the empirical formula.
In another example, a hydrate sample of MgxCly·nH2O is given. The mass of the water molecules would again be easily determined by heating. Using some solubility rules, chloride is then precipitated with silver ion, Ag+, to form AgCl(s). Once the mass of AgCl(s) is found, the moles of Cl- are determined using the molar mass of AgCl(s) and then converted to grams of Cl-. This would allow us to determine the mass of Mg in the sample followed by the empirical formula.
Determining an empirical formula is at the center of identifying the formula of the actual molecule. From pharmaceuticals to forensics, determination of a molecular formula is key to identifying an unknown compound, which means taking the empirical formula to the next step. Typically, the determination of an empirical formula is coupled with elemental analysis to obtain elemental weight percent information. From these data, the molar ratios are calculated and the empirical formula is determined. We can determine the mass of molecule using another analytical tool, like a mass spectrometer. Then, the ratio between the molecular mass and empirical mass is calculated to determine the true molecular formula.
Skip to...
Videos from this collection:

Now Playing
Determining the Empirical Formula
General Chemistry
179.9K Views

Common Lab Glassware and Uses
General Chemistry
653.1K Views

Solutions and Concentrations
General Chemistry
272.9K Views

Determining the Density of a Solid and Liquid
General Chemistry
554.6K Views

Determining the Mass Percent Composition in an Aqueous Solution
General Chemistry
383.0K Views

Determining the Solubility Rules of Ionic Compounds
General Chemistry
141.0K Views

Using a pH Meter
General Chemistry
344.2K Views

Introduction to Titration
General Chemistry
423.3K Views

Ideal Gas Law
General Chemistry
78.0K Views

Spectrophotometric Determination of an Equilibrium Constant
General Chemistry
158.2K Views

Le Châtelier's Principle
General Chemistry
263.9K Views

Freezing-Point Depression to Determine an Unknown Compound
General Chemistry
160.4K Views

Determining Rate Laws and the Order of Reaction
General Chemistry
195.7K Views

Using Differential Scanning Calorimetry to Measure Changes in Enthalpy
General Chemistry
44.4K Views

Coordination Chemistry Complexes
General Chemistry
91.2K Views
Copyright © 2025 MyJoVE Corporation. All rights reserved