Method Article
Parameterizing V-notch Weir Equations for Flow Monitoring in a Drainage Control Structure
In This Article
Summary
This protocol describes methods for obtaining weir flow equation coefficients for a V-notch weir within a drainage control structure using (1) a laboratory calibration procedure and (2) an online tool, the "Weir Flow Equation Coefficients Calculator", developed by the authors. These methods apply to flows contained within the V-notch and overtopping flows.
Abstract
Accurate estimation of drainage discharge (flow rate or water volume per unit time) is essential for calculating nutrient loads delivered to surface water and assessing the performance of edge-of-field conservation practices. Determining drainage flow rates requires monitoring the head in a drainage control structure and applying an appropriate equation for the weir installed in the structure. Previous studies that developed calibrated equations for weirs in control structures have produced different equations (i.e., different weir flow equation coefficients) for the same type of weir and control structure size. This study describes procedures for setting up experimental water flow rate and head measurements within a drainage control structure and developing a weir equation for flows contained within a V-notch weir. Additionally, the procedure for obtaining a weir equation for overtopping flows -- when the water level exceeds the top of the V-notch weir -- is outlined. This process involves using the weir equation developed in the laboratory for a V-notch weir and an online tool, the "Weir Flow Equation Coefficients Calculator", developed by the authors. The online tool can also generate weir flow equation coefficients for both V-notch-contained and overtopping flows across various sizes of Agri Drain water control structures, even when users lack site-specific flow rate vs. head relationship.
Introduction
In evaluating the performance of edge-of-field conservation practices that reduce nutrient loss from subsurface drainage water (saturated buffers, bioreactors, and drainage water management), it is important to accurately determine drainage discharge and nutrient concentrations. Standard methods are readily available for accurately determining nutrient concentrations (e.g., nitrate), such as laboratory methods from water samples1 or sensors for continuous monitoring of nutrients2,3. Determination of drainage flow rates in edge-of-field conservation practices, on the other hand, is not as straightforward as with nitrate concentration and usually involves the use of a drainage water level control structure4,5. A drainage water level control structure is used in an edge-of-field conservation practice with the dual purpose of controlling the upstream water head and monitoring the discharge flow rates with the use of weirs. Important components to consider while determining drainage flow rates include the size of the drainage control structure, the type of weir used in the structure, flow conditions within the structure, accurate measurement of heads, and the use of appropriate weir equations to convert heads to discharge flow rates. While these structures are generally limited to artificially "tile" drained lands, drained agricultural land exceeds 21 million ha in the US6 and impacts nutrient loads to streams and rivers7.
Determination of flow rates using weirs is a common practice, and standard procedures and calibration equations are available for flows in open channels8. However, only a limited number of studies have developed calibration equations for weirs in a drainage control structure5,9,10,11,12,13,14,15,16,17. Chun and Cooke10 pioneered the development of calibration equations for rectangular weirs for various sizes of control structures. Following Chun and Cooke10, Christianson et al.9 developed a single calibration equation for a 45° V-notch weir in 15 cm (6 in) and 25 cm (10 in) control structures with weirs placed at different heights from the bottom of the structures. Shokrana and Ghane14 provided a different flow equation for a 45° V-notch weir in a 25 cm (10 in) structure from Christianson et al.9, when the weir was placed about 30 cm above the bottom of the structure. The authors attributed the difference in the two calibration equations to the use of a calibrated weighing device for measuring flow rates, suggesting that a unified procedure for obtaining flow rates, heads, and the calibration equations for weirs for flows contained within the V-notch in control structures is lacking.
In our recent study11, we developed calibration equations for 45° V-notch weirs in Agri Drain control structures based on their size (15cm [6 in], 20 cm [8 in], and 30 cm [12 in]) for flows contained within the V-notch and overtopping flows (i.e., when the head exceeds the top of a V-notch weir). While the calibration equation for flows contained within the V-notch is a simple power relationship between head (h) and flow rate (Q, equation 1)5,9,11,13,14, overtopping flows require the use of a compound weir equation, which is more complicated (i.e., has more terms) because it combines equations for a V-notch weir and a rectangular weir (equation 2)11.
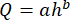 (1)
(1)
where, Q = flow rate, h = head, and a and b = weir coefficients whose values depend on the shape of the weir, the size of the control structure, the flow conditions, and the units for Q and h.
 (2)
(2)
where, Q = flow rate, h = the total head, h1 = head above the top of V-notch weir (i.e., in the rectangular weir above the V-notch), W = width of the rectangular weir section above the top of V-notch weir, Wv = top width of the V-notch weir, a and b = weir coefficients for the V-notch weir, and a1 and b1 = weir coefficients for the rectangular section above the V-notch weir.
The adoption of a smart drainage system with sensors to monitor and automatically adjust the water levels in a control structure using remotely controlled adjustable gate valves is increasing in edge-of-field conservation practices in the US. Most of these systems, which were originally designed to use only a simple power function, cannot accommodate the compound weir equation (equation 2) for the overtopping flows and demand the development of unique flow equations of the form shown by equation (1) for each drainage control structure for overtopping flows. Additionally, users involved in measuring and monitoring flow rates and nutrient losses may find it cumbersome to use equation (2) for overtopping flows due to the requirement for more parameters. To overcome these challenges, we developed the "Weir Flow Equation Coefficients Calculator" tool. This online tool (available at: https://www.ars.usda.gov/midwest-area/ames/nlae/docs/tools-available-from-nlae/) provides weir flow equation coefficients for standard sizes of drainage control structures (Table 1) for a 45° V-notch weir. More importantly, the tool calculates weir flow equation coefficients for overtopping flow and consists of only two parameters, as presented in equation (1), based on the size and dimensions of the control structure provided by the user. When the appropriate equipment is available to measure site-specific flow rate vs. head relationships, the tool also accepts a calibration equation for flows contained within the V-notch weir as user input and calculates the weir flow equation coefficients for overtopping flows. Thus, this protocol describes the setup for flow measurement in a drainage control structure and a universal method for the development of a site-specific calibration equation. Next, the use of the tool "Weir Flow Equation Coefficients Calculator" for calculating weir flow equation coefficients for flows contained within the V-notch and overtopping flows is described. Finally, monitoring and maintenance of flows in a drainage control structure is described, which will facilitate accurate estimation of drainage flow rates using the weir equations obtained from the tool.
Protocol
The details of the consumables and equipment used in this study are listed in the Table of Materials.
1. Obtaining weir flow equation coefficients for flows contained within the V-notch by laboratory calibration in a drainage control structure
- Setting up the drainage control structure for a calibration experiment
- Connect the inlet section of the control structure to the pump assembly (consisting of a pump, a flow meter, and the necessary pipes, fittings, and valves) and the outlet section to the drainage pipe (see Figure 1). Ensure to seal additional outlets from the control structure, if any.
- Measure the top width (Wv, Figure 2A) and depth (D, Figure 2B) of the V-notch weir.
- Measure the distance (width, W) between the tracks (Figure 3A) where the stoplogs (boards used for maintaining the water level depth in the control structure, Figure 3B) and V-notch weir fit in the drainage control structure.
- Insert the stoplogs between the tracks one at a time. Ensure the bottom stoplog sits tightly on the bottom of the control structure so that there is no leakage from beneath the stoplog.
- Stack additional stoplogs above the existing ones in the control structure until the desired height is reached. Ensure that the stoplogs are set tightly and no leakage occurs between the stoplogs and the rails.
NOTE: The total height of the stoplogs should be greater than the opening of the inlet pipe and correspond to the design depth of the water table in the saturated buffer, bioreactor, or controlled drainage system. The setup in the laboratory for the calibration experiment should best represent the flow conditions in the field for accurate flow rate estimations. - Place the weir plate firmly above the top stoplog.
- Measure the inner height of the control structure (D1) and the distance between the top of the control structure and the top of the V-notch weir (D2) using a measuring tape which has its zero-marking flushed with the edge of the tape (see Figure 4A).
- Calculate the height of the V-notch crest from the bottom of the control structure (D3) as: D3 = D1- D2- D, where D is the depth of V-notch obtained from step 1.1.2.
NOTE: Do not calculate the height of the V-notch crest from the bottom of the control structure as the sum of the heights of stoplogs used as the rubber gasket around the stoplogs increases the combined height of stoplogs. - Firmly connect the manometer tube/stilling well (used for determining the water level in the control structure) to the control structure. Affix a tape measure to the outside wall of the control structure next to the manometer with the "zero" value corresponding to the base of the interior of the control structure (see Figure 4B).
- Measuring flow rates and head
- Start the pump and allow water to fill the inlet chamber and subsequently flow through the weir.
- Select a flow rate of about 1-1.5 L.s-1 (15-25 gpm) by adjusting the valve in the pump assembly and monitoring the display on the flow meter.
- Allow sufficient time for the flow to stabilize, usually at least 3 min. The corresponding head should be at least 6 cm (>2.4 in) so that the flow nappe does not stick to the weir plate8. Increase the flow rate if the head is less than 6 cm (2.4 in) (Figure 5A).
- Once the flow is stable, record the flow rate reading from the display on the flow meter. Take 8-10 consecutive readings within 2 min and calculate the average flow rate (Q) (Figure 5B).
- Measure the height of water in the stilling well/manometer tube. If the water level in the stilling well/manometer tube fluctuates, take the readings for the high and low levels of water during a period of at least 30 s and obtain the average value for the height of water above the bottom of the structure (D4) (Figure 5C).
- Calculate the head (h) as: h = D4- D3.
- Increase the flow rate by approximately 1-1.3 L.s-1 (15-20 gpm) and wait for the flow rate to stabilize.
- Obtain the flow rate and corresponding head as described in steps 1.2.3-1.2.6.
- Increase the flow rate at least 5 more times until the water level reaches near the top of the V-notch and repeat procedures 1.2.3-1.2.6 for each increase in the flow rate.
- Developing the weir calibration equation for flows contained within the V-notch
- In an Excel spreadsheet, enter the values of the head (h, cm) and the corresponding flow rates (Q, L.s-1) through the V-notch weir.
NOTE: An Excel spreadsheet consisting of measured flow rates and heads in a 20 cm (8 in) control structure is provided as Supplementary File 1. - Plot the measured flow rates (Q, Y-axis) against the measured head (h, X-axis) and fit a power function as in equation (1). Select the data range in the spreadsheet, then click Insert > Charts > Scatter. Right-click on the data points in the chart, then click Add Trendline > Power > Display Equation on chart > Display R-squared value on chart.
- Check the goodness-of-fit of the power function both visually and by using R2 value (Figure 6). This is the weir equation for flow contained within V-notch for the control structure.
- In an Excel spreadsheet, enter the values of the head (h, cm) and the corresponding flow rates (Q, L.s-1) through the V-notch weir.
2. Using "Weir Flow Equation Coefficient Calculator" for obtaining weir coefficients for flows contained within the V-notch and overtopping flows
NOTE: The following steps require the use of the "Weir Flow Equation Coefficient Calculator" tool available at https://www.ars.usda.gov/midwest-area/ames/nlae/docs/tools-available-from-nlae/.
- Weir flow equation coefficients for overtopping flows using own calibration equation
- Open the link https://www.ars.usda.gov/midwest-area/ames/nlae/docs/tools-available-from-nlae/ and click on “Weir Flow Equation Coefficients Calculator” on the webpage. This step will open the tool in a new browser (Supplementary Figure 1).
- Select the unit between Metric (SI) and US Customary units using the radio buttons (Supplementary Figure 2).
- In the section Size of control structure, leave the selection to the default value of 6-in (15-cm) as the user provides their own weir equation coefficients.
- Click on Yes as the response to "Do you have your own calibration equation?" and enter the values of the parameters a and b corresponding to the equation for flows contained within the V-notch, Q = ahb and obtained from step 1.3.2 (Supplementary Figure 2).
- Enter the values of the weir specifications in the Inputs section of the tool. The inputs include the width between the tracks (W), depth of V-notch (D), and width of V-top (Wv). Refer to the figure on the right for the symbols (Supplementary Figure 2).
- Get the values of coefficients for overtopping flows from the table Coefficients for V-notch weir equation, Q = ahb, and the plot of flow rate against the head from the Results section of the tool (Supplementary Figure 2).
- Weir flow equation coefficients for flows contained within the V-notch and overtopping flows using the tool
- Open the tool as described in section 2.1.1. and select the unit and the size of the control structure using the radio buttons (Supplementary Figure 3).
- Click on No as the response to "Do you have your own calibration equation?"
- Enter the values of the weir specifications as described in section 2.1.5.
- Get the values of the coefficients for flows with V-notch weir, and overtopping flows from the table and the plot of flow rate vs. flow depth relative to the V-notch crest from the Results section of the tool (Supplementary Figure 3).
3. Flow monitoring and maintenance in drainage control structures
- Verification of water levels in a drainage control structure
NOTE: Ensuring that the flow levels obtained from the sensors are correct is crucial for accurate estimation of flow rates, which is achieved by comparing sensor readings with manual measurements through regular field visits.- Using a tape measure, measure the distance of the water level from the top of the control structure in the inlet chamber near the location of the sensor within the chamber.
- To take the measurement, lower the tape measure into the control structure until it just touches the meniscus of the water level. Take this measurement 1-3 times and record the distance.
- To obtain the record of the sensor reading, open the control panel box and record the number corresponding to the water level reading (Figure 7).
NOTE: Depending on the settings of the control panel, the screen may display the water level measurement from the top or the bottom of the drainage control structure. - To compare the manual measurement with the sensor reading corresponding to a value of water depth from the bottom of the control structure, subtract your manual measurement from the interior height of the control structure and compare it with the sensor reading.
- If there is a discrepancy between the measured and sensor values, check for sources of errors, including the presence of sediments/debris within the control structure, unaccounted changes in weir height resulting from weir management, dislocation of the sensors, and malfunctioning of the sensors and correct accordingly.
- Obtaining time series of flow rates
NOTE: A spreadsheet consisting of example data from a saturated buffer site (BC-2)18 and the procedure for obtaining time series of flow rates using the weir equation coefficients from the tool is described in sections 2.2.1-2.2.4, and is presented in Supplementary File 2.- Download the time series of flow level data from the respective datalogger or server associated with the drainage system.
- Using the time series flow level data, calculate the head.
- Determine the flow condition, i.e., flow contained within the V-notch (h ≤ D) or overtopping flow (h > D) by comparing head (h) with the depth of V-notch (D) for each time record.
- Calculate the drainage flow rate for the flow condition using the weir equation (1) with weir flow equation coefficients obtained from the "Weir Flow Equation Coefficient Calculator" tool (as described in section 2.1. or 2.2.).
- In an edge-of-field conservation practice equipped with a smart drainage system, get the time series of flow rates directly from the dashboard of the respective website (Figure 8).
Results
The calibration of a stainless-steel 45° V-notch weir was performed in 15 cm (6 in), 20 cm (8 in), and 30 cm (12 in) Agri Drain control structures for flows contained within the V-notch11. The measured values of flow rates and heads and the weir equations obtained by fitting a power function (equation 1) to the measured data for flows contained within the V-notch, as described in the protocol, are presented in Figure 9A-C. These weir flow equation coefficients for flows contained within the V-notch are included in the "Weir Flow Equation Coefficient Calculator" tool. Weir equations for the overtopping flows presented in Figure 9A-C were obtained from the "Weir Flow Equation Coefficient Calculator" tool. The errors in estimating flow rates were calculated by comparing the calculated flow rates with the measured flow rates using root-mean-square error (RMSE, equation 3) and percentage bias (PBIAS, equation 4) as19:
 (3)
(3)
 (4)
(4)
where, yi,meas = measured flow rate at flow depth i, yi,pred = predicted flow rate at head i, n = total number of head-flow rate measurements.
Figure 9A-C shows that the use of the weir equation obtained through a dedicated calibration procedure for flows contained within the V-notch can produce estimated flow rates with high accuracy (RMSE < 0.20 L.s-1 and PBIAS < 1%). The flow rates for the overtopping flow estimated using weir equation coefficients from the "Weir Flow Equation Coefficient Calculator" tool were similar to the measured values of flow rates with RMSE < 2.6 L.s-1 and the absolute value of PBIAS < 3.5%. These results confirm that our "Weir Flow Equation Coefficient Calculator" tool can be used to obtain weir flow equation coefficients for V-notch weirs in various sizes of control structures. These weir flow equations coefficients (a and b) can be used to calculate flow rates using a simple equation, Q = ahb, with high accuracy for flows both contained within the V-notch weir and for overtopping flows.
In addition to providing weir flow equation coefficients for 15 cm (6 in), 20 cm (8 in), and 30 cm (12 in) control structures, the tool also provides weir flow equation coefficients for 10 cm (4 in), 25 cm (10 in), and 38 cm (15 in) control structures. Since a 10 cm (4 in) and a 15 cm (6 in) Agri Drain control structure have equal widths between the tracks (Table 1), the weir flow equation coefficients do not vary for a 45° V-notch weir in the two structures. As the width between the tracks increases with the size of the control structure and thus the degree of flow contraction through the weir, the weir flow equation coefficients change with the variation in the size of the control structure, as shown in Figure 9A-C. However, once the flow has fully contracted, a further increase in the width does not affect the relationship between flow rate and depth8. For rectangular weirs in Agri Drain control structures, Chun and Cooke10 reported that the weir flow equation coefficients do not change for structures greater than 20 cm (8 in). For a 45° V-notch weir, we expect that the weir flow equation coefficients for control structures greater than 30 cm (12 in) do not vary for flows contained within the V-notch. Therefore, for a 38 cm (15 in) control structure, the tool uses the same weir flow equation as a 30 cm (12 in) control structure for flows contained within the V-notch. The weir flow equation for a 25 cm (10 in) control structure for flows contained within the V-notch was obtained by interpolating flow rates corresponding to various flow depths between 20 cm (10 in) and 30 cm (12 in) control structures and a fitting power function (equation 1) to the head and flow rates (see Supplementary Figure 4 for the calibration curve).
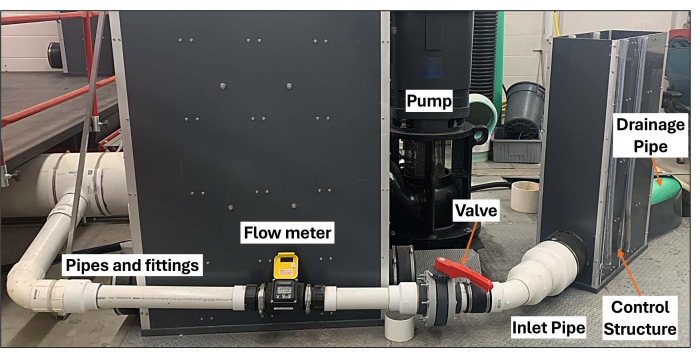
Figure 1: Setup of the drainage control structure for the V-notch weir calibration experiment. Please click here to view a larger version of this figure.
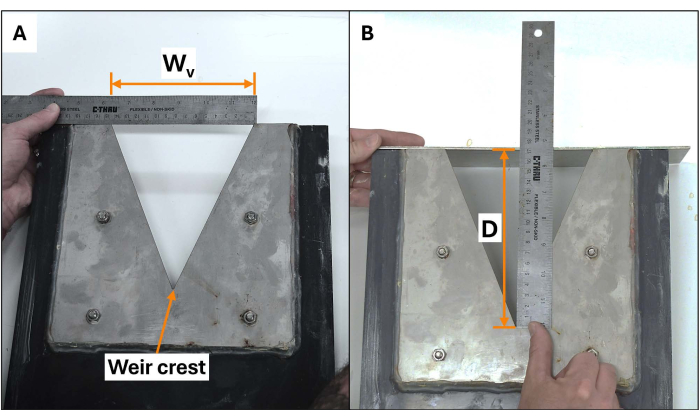
Figure 2: Specifications of the weir used in a drainage control structure. (A) Measurement of top width (Wv) and (B) depth of V-notch weir (D). Please click here to view a larger version of this figure.
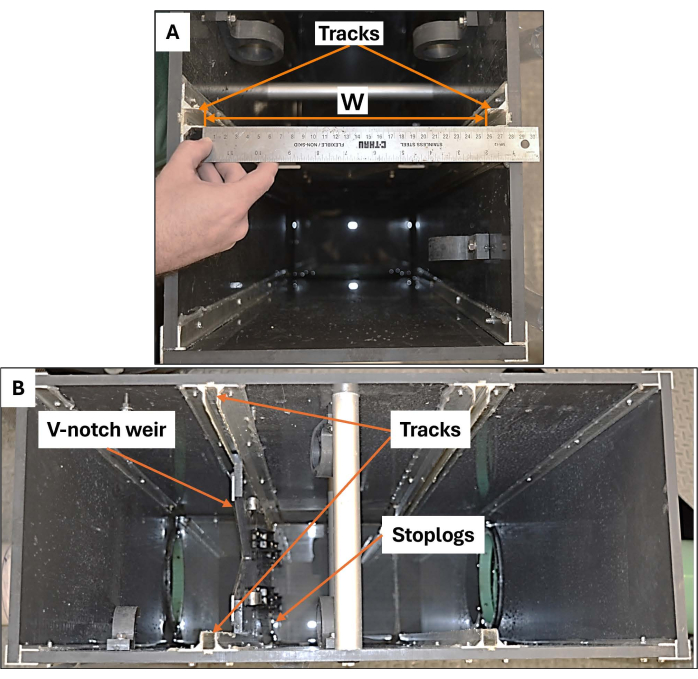
Figure 3: Measurements and positioning of the V-notch weir in the drainage control structure. (A) Obtaining the width of the weir (W) as the distance between the tracks in a drainage control structure. (B) Placement of stoplogs and the V-notch weir in the drainage control structure. Please click here to view a larger version of this figure.

Figure 4: Measurements and instruments required for determining the head. (A) Obtaining the inner height of the control structure (D1) and the distance of V-notch top from the top of the structure (D2) for the determination of the height of the V-notch crest from the bottom of the control structure (D3). (B) Setup of manometer and a tape measure on the outside the control structure for measuring the head (h). Please click here to view a larger version of this figure.
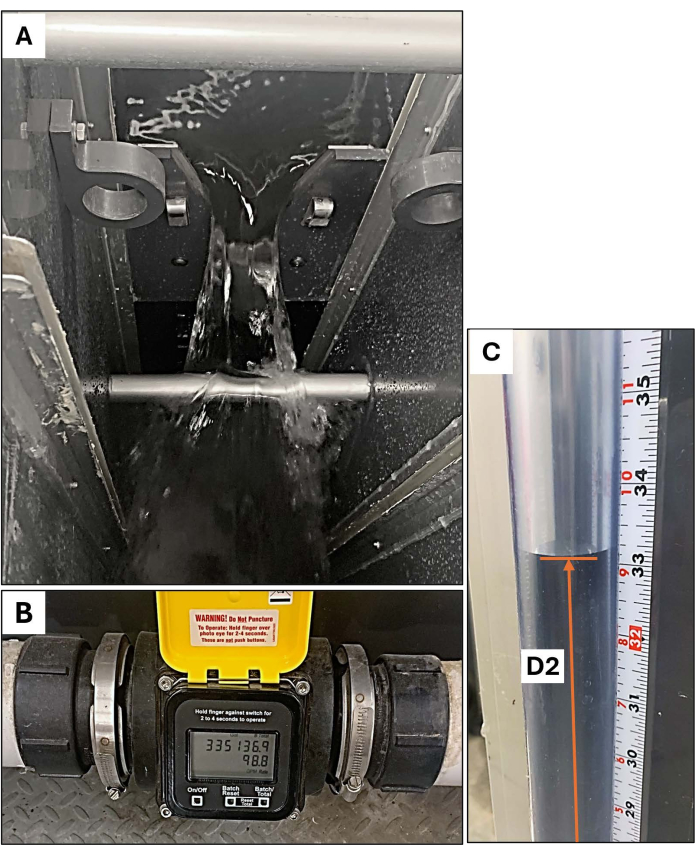
Figure 5: Measurements of flow rate and head. (A) Water flow through the V-notch weir. (B) Flow rate reading on the flow meter connected to the pump and the control structure. (C) Measurement of water level height corresponding to the flow rate shown in Figure 5B. Please click here to view a larger version of this figure.

Figure 6: Calibration equation. Obtaining the calibration equation using the measured head and flow rates for a V-notch weir in a 20.32 cm (8 in) drainage control structure using a power function in an excel spreadsheet. Please click here to view a larger version of this figure.
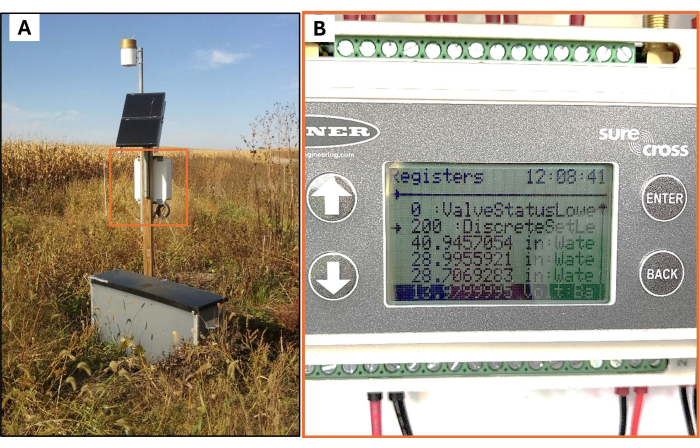
Figure 7: Flow monitoring in the field. (A) A drainage control structure installed in a saturated buffer site. (B) Display of control panel box for obtaining sensor readings for sensors equipped in the drainage control structure (orange rectangle in Figure 7A). Please click here to view a larger version of this figure.
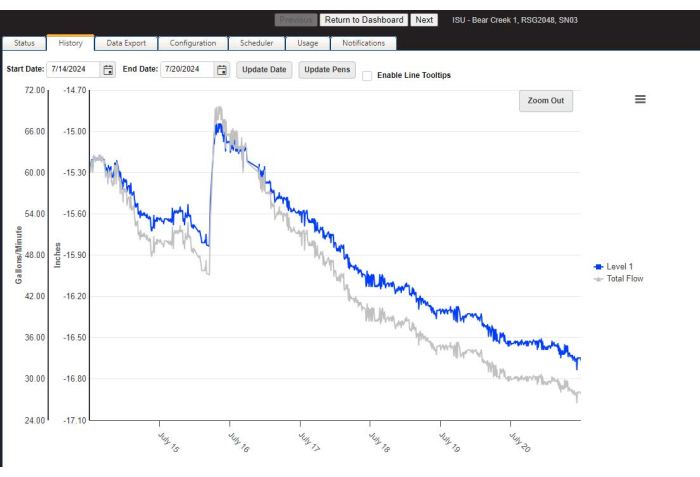
Figure 8: Screenshot of the time series of flow levels and flow rates from an Agri Drain smart drainage system installed in a saturated buffer in central Iowa. Please click here to view a larger version of this figure.

Figure 9: Plots of measured flow rates vs heads for various sizes of control structures. (A) 15.24 cm (6 in) control structure, (B) 20.32 cm (8 in) control structure, and (C) 30.48 cm (12 in) control structure. The weir equations for flows with V-notch and for overtopping flows are provided within each subplot. The weir flow equations for flows contained within the V-notch were obtained from Katuwal et al.11 and for overtopping flows using the "Weir Flow Equation Coefficients Calculator" tool. Abbreviation: RMSE = Root Mean Square Error; PBIAS = Percentage bias. Please click here to view a larger version of this figure.
| Size of control structure, cm (inch) | Width of stoplog between the rails (W), cm (inch) |
| 10 (4) | 16.19 (6.375) |
| 15 (6) | 16.19 (6.375) |
| 20 (8) | 25.4 (10) |
| 25 (10) | 31.43 (12.375) |
| 30 (12) | 36.51 (14.375) |
| 38 (15) | 46.67 (18.375) |
Table 1: Specifications of the control structures based on their size.
Supplementary Figure 1: Screenshot of the graphical user interface of the online tool "Weir Flow Equation Coefficients Calculator". The tool is available at https://www.ars.usda.gov/midwest-area/ames/nlae/docs/tools-available-from-nlae/. Please click here to download this File.
Supplementary Figure 2: Illustration of the procedure for obtaining weir equation coefficients for overtopping flow using the tool "Weir Flow Equation Coefficients Calculator" when the user has their own calibration equation for the V-notch weir. Each sequential step is marked with the step number and presented within a green rectangle. Sections of tools not included within a green rectangle indicate steps that should be skipped. Please click here to download this File.
Supplementary Figure 3: Illustration of the step-by-step procedure for obtaining weir equation coefficients using the tool "Weir Flow Equation Coefficients Calculator" when the user does not have their own calibration equation for the V-notch weir. Each sequential step is marked with the step number and presented within a green rectangle. Sections of tools not included within a green rectangle indicate steps that should be skipped. Please click here to download this File.
Supplementary Figure 4: Calibration curve for a 45° V-notch weir in a 25 cm (10 in) control structure and its comparison with previous studies. This figure is adapted from Katuwal et al.11. Please click here to download this File.
Supplementary File 1: Example dataset consisting of measured flow rates and heads in a 20 cm (8 in) control structure for obtaining calibration equation for a V-notch weir presented in Figure 6. Please click here to download this File.
Supplementary File 2: Spreadsheet consisting of an example dataset from a saturated buffer site (BC-2)18 and the procedure for obtaining time series of flow rates using the weir equation coefficients from the online tool. Please click here to download this File.
Discussion
The protocol (step 1) describes a method for developing a calibration equation for a 45° V-notch weir in an Agri Drain drainage control structure. However, the method can be adapted for developing calibration equations for different types of weirs, such as a rectangular weir, a trapezoidal weir, or a V-notch weir with various angles in any drainage control structure. During the calibration procedure, it is particularly important that accurate heads are obtained, especially at greater flow rates when the water level inside the control structure tends to be more turbulent. The water level should be measured within a stilling well or a manometer tube. Attaching a measuring tape close to the manometer tube/stilling well enables checking for the water levels and simultaneous measurements for water level height, thus reducing errors in head measurements. If not already present in the drainage control structure, modification of the control structure may be necessary by adding a manometer or the stilling well positioned at the farthest location from the weir crest. If a water level measuring device/sensor is used for measuring the water level height, the measurements obtained from the devices should be verified with manual measurements.
For accurate flow rate measurements using a flow meter, it is important that the straight pipe requirements for upstream and downstream from the flow meter are met according to the American National Standards Institute/Hydraulic Institute (ANSI/HI) or the manufacturers' recommendations for the flow meter used.
While the protocol described in step 1 can also be used to develop a calibration equation for overtopping flows in the laboratory by measuring heads and flow rates (4-6 measurements for overflows, steps 1.2.3-1.2.6) and fitting a power function (steps 1.3.1-1.3.3), it is not commonly practiced. One of the factors is the dependence of the flow coefficients not only on the angle of the V-notch but also on the top width of the V-notch weir (Wv), which varies with the depth of the V-notch weir (D)11.
The weir flow equation coefficients that are obtained using the "Weir Flow Equation Coefficient Calculator" tool were based on laboratory calibrations performed in a two-chamber control structure11. Therefore, the coefficients are valid only for a sharp-crested 45° V-notch weir in a two-chamber control structure or for a weir present in the inlet chamber of a control structure with more than two chambers. The use of the same weir equation for the downstream weir (second weir) in a control structure with more than two chambers may result in underestimation of flow rates, especially at higher heads because of increased flow velocities/turbulence inside the structure unless flow dampening structures11,20 are installed before the second weir. It should also be noted that the weir flow equation coefficients are for free flow conditions, i.e., when the water level downstream of the weir is below the V-notch weir crest. The equations may result in an overestimation of flow rates for submerged conditions. The weir equations have not yet been validated for estimating flows in control structures where other flow openings, such as orifices12 are present in addition to weirs.
The "Weir Flow Equation Coefficient Calculator" tool provides weir flow equation coefficients for a 45° V-notch weir in standard sizes of Agri Drain drainage control structures21 and V-notch position of at least 60 cm (2 ft) above the bottom of the control structure, and therefore, use of the tool for control structures whose dimensions deviate from the size of the structures presented in Table 1 and different weir position may result in inaccurate flow rates. In these cases, the use of site-specific weir equations or weir equations from previous studies developed under similar flow conditions is advised. However, as the protocol describes, the tool can utilize users' site-specific weir calibration equation as input and, therefore, can easily accommodate V-notch weirs of different angles or custom-made Agri Drain control structures and provide weir flow equation coefficients for overtopping flows. It must also be noted that the weir equation coefficients for a 25 cm (10 in) structure are obtained by interpolation between 15 cm (6 in) and 30 cm (12 in) control structure, and the 38 cm (15 in) control structure uses the same weir equation as a 30 cm (12 in) control structure. Additionally, the weir equation coefficients for overtopping flows have been validated for heads up to 38 cm (15 in) only.
Disclosures
All opinions expressed in this paper are the authors' and do not necessarily reflect the policies and views of USDA, DOE, or ORAU/ORISE. Any use of trade, firm, or product names mentioned in this article is for descriptive purposes only and does not imply recommendations or endorsement by the US Department of Agriculture. USDA is an equal opportunity provider and employer.
Acknowledgements
The authors acknowledge the assistance of Shane Svoboda in taking photographs used in this article. This work was supported in part by an appointment to the Agricultural Research Service (ARS) Research Participation Program administered by the Oak Ridge Institute for Science and Education (ORISE) through an interagency agreement between the US Department of Energy (DOE) and the US Department of Agriculture (USDA). ORISE is managed by ORAU under DOE contract number DE-SC0014664. This research was a contribution from the Long-Term Agroecosystem Research (LTAR) Network. LTAR is supported by the United States Department of Agriculture, Agricultural Research Service.
Materials
| Name | Company | Catalog Number | Comments |
| Flow meter | Banjo Corp | MFM300 | Electromagnetic flowmeter, accuracy within ± 2-3% for flow rates between 0.88 and 42.3 L.s-1 (14 to 670 gpm), and operating temperature range of -12 to 54 °C |
| Pipe and fittings | N/A | N/A | The pipe length and fittings should be as necessary but fulfill the miniumum straigth pipe requirements for upstream and downstream of flow meter according to manufacturer's recommendation. |
| Pump | US Motors | 15706017-100 | Model 15411 60 HP 3 Phase VFD driven |
| Stoplogs | Agri Drain | 700320 (5 in. stoplog), 700321 (7 in. stoplog), 700333 (7 in. bottom stoplog with bottom gasket) | Three types of PVC stoplogs of heights 5 inch, 7 inch, and 7 inch bottom stoplog with bottom gasket |
| Tape Measure | Stanley PowerLock | 33-158 | 5m/16' tape measure |
| V-notch weir | Agri Drain | 701132 | Stainless steel 45° (3 mm thickness, V-notch depth of 17.145 cm) notched weir with PVC carrier (13 mm thickness) |
| Water Level Drainage Control Structure | Agri Drain | 3CINLINE06X08PVC | A 3-chambered inline water level control structure with nominal size of 20.32 cm (8 inch) and V-notch weir present in the first gate (Stoplogs included in purchase) |
References
- American Public Health Association. . Standard methods for the examination of water and wastewater. , (2023).
- Alahi, M. E. E., Mukhopadhyay, S. C. Detection methods of nitrate in water: A review. Sens Actuators A Phys. 280, 210-221 (2018).
- Arenas Amado, A., Schilling, K. E., Jones, C. S., Thomas, N., Weber, L. J. Estimation of tile drainage contribution to streamflow and nutrient loads at the watershed scale based on continuously monitored data. Environ Monit Assess. 189 (9), 426 (2017).
- Christianson, L., et al. Performance evaluation of four field-scale agricultural drainage denitrification bioreactors in Iowa. Trans ASABE. 55 (6), 2163-2174 (2012).
- Jaynes, D. B., Isenhart, T. M. Reconnecting tile drainage to riparian buffer hydrology for enhanced nitrate removal. J Environ Qual. 43 (2), 631-638 (2014).
- National Agricultural Statistics Service (NASS). . 2022 census of agriculture. , (2024).
- Dinnes, D. L., et al. Nitrogen management strategies to reduce nitrate leaching in tile-drained midwestern soils. Agronomy J. 94 (1), 153-171 (2002).
- United States Bureau of Reclamation (USBR). . Water measurement manual. , (2001).
- Christianson, L. E., et al. Calibration of stainless steel-edged v-notch weir stop logs for water level control structures. App Eng Agric. 35 (5), 745-749 (2019).
- Chun, J. A., Cooke, R. A. Technical note: Calibrating agri drain water level control structures using generalized weir and orifice equations. App Eng Agric. 24 (5), 595-602 (2008).
- Katuwal, S., et al. Calibration of v-notch and compound weirs for subsurface drainage water level control structures. App Eng Agric. 40 (4), 453-463 (2024).
- Maxwell, B. M., Cooke, R. A., Christianson, R. D., Christianson, L. E. Stage-discharge relationships of drawdown plates for denitrifying woodchip bioreactors. App Eng Agric. 37 (6), 1023-1029 (2021).
- Partheeban, C. "Demonstrating the Effectiveness of Nitrate-nitrogen Removal of Denitrifying Bioreactors in South Dakota for Improved Drainage Water Management". Electronic Theses and Dissertations. 635, (2014).
- Shokrana, M. S. B., Ghane, E. An empirical v-notch weir equation and standard procedure to accurately estimate drainage discharge. App Eng Agric. 37 (6), 1097-1105 (2021).
- Utt, N., Jaynes, D., Albertsen, J. Demonstrate and evaluate saturated buffers at field scale to reduce nitrates and phosphorus from subsurface field drainage systems. Report No. CIG Final Report to NRCS and FSA. , (2015).
- Napierała, M. Application of simple crested weirs to control outflows from tiles drainage. Water. 15 (18), 3248 (2023).
- El Hattab, M. H., Mijic, A., Vernon, D. Optimised triangular weir design for assessing the full-scale performance of green infrastructure. Water. 11 (4), 773 (2019).
- Jaynes, D. B., Isenhart, T. M. Performance of saturated riparian buffers in Iowa, USA. J Environ Qual. 48 (2), 289-296 (2019).
- Moriasi, D. N., Gitau, M. W., Pai, N., Daggupati, P. Hydrologic and water quality models: Performance measures and evaluation criteria. Trans ASABE. 58 (6), 1763-1785 (2015).
- Johnson, G., et al. Effectiveness of saturated buffers on water pollutant reduction from agricultural drainage. J Nat Resour Agric Ecosyst. 1 (1), 49-62 (2023).
- . Inline water level control structures Available from: https://www.agridrain.com/shop/c85/manual-water-level-control-structures/p901/inline-water-level-control-structures/ (2024)
Reprints and Permissions
Request permission to reuse the text or figures of this JoVE article
Request PermissionExplore More Articles
This article has been published
Video Coming Soon
Copyright © 2025 MyJoVE Corporation. All rights reserved